New Variable For Effective Demand Limit… Part 2
<< Read: Variables For An Effective Demand Limit To The Business Cycle Part 1
For those nutty readers that follow the effective demand story that I present on Angry Bear, today, the first day of 2015, marks a breakthrough. I just posted yesterday a model to expand the variables for assessing the effective demand limit upon production. Today a new variable is added to the mix with interesting results…
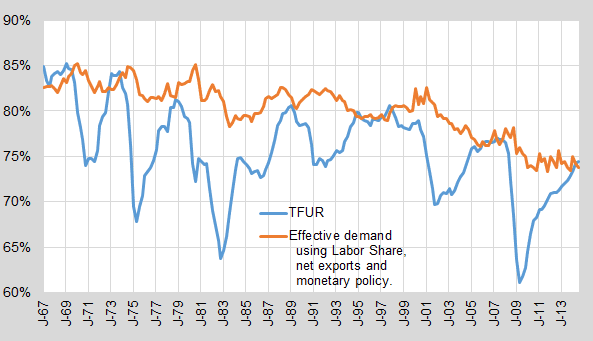
Here is the graph that ended the previous post…
Graph #1
We see an effective demand limit (orange line) upon the production cycle of utilizing labor and capital (blue line). The problem remained that there were still gaps that had to be filled. Let me point them out.
Graph #2
If I increased the coefficient on the variable for monetary policy (ED-FF), I could close the gaps at 1981 and 2000, but a gap would then open up at 1979. Like this…
Graph #3
Would it be possible to close the gaps in graph #2 without opening up other gaps that have already closed? It almost seems impossible or super complicated?
Well, let’s go back and look at the stagflation of the late 70′s. What happened? The abrupt rise in commodity prices produced a price supply shock which affected demand to the extent that production had to be cut. As the effective demand model is designed to show the times when production is cut, it has to incorporate the effects of supply shocks too.
So I incorporate another variable into the limit function. Let me first place the new graph which incorporates this new variable.
Graph #4
Let’s analyze this graph first… You will see that the huge gap in 1981 closed while the gap in 1979 did not open. Closing that gap in 1981 is a big accomplishment in understanding the effective demand limit.
You will also see that the gap in 2000 closed. This also was an important gap to close in order to coincide effective demand with the beginning of the 2001 recession. You will also see that the gap from 1995 to 1998 was stable. Maintaining the stability of this gap in the model was important because the economy was riding the natural limit of real GDP in a stable way during those years. Production increases through 1997, but fell back to below effective demand.
We can also see that effective demand was biting into production in 1988 setting up the eventual recession which started in 1990.
So, what variable did I include? It is a variable for supply shocks that trigger stagflation… The variable is the Consumer Price Index of all items, yoy%. (link) Simply put, I incorporated inflation. The idea was to see how much a forced rise in prices to consumers would affect the effective demand limit.
Graph #5
Inflation had large jumps in the 70′s. Those jumps might close that gap that opened up in 1979 (see graph #3). The idea is that a quick jump in prices will take the consumer by surprise to the extent that they will lower their demand for production. If this jump happens close to the top of a business cycle, it can lower effective demand to where it falls upon expanding production and cuts short the business cycle expansion.
The equation of the limit function in graph #4 is…
Limit function, L = 0.765LS + 10NX/rGDP – 55CPIall + 80(ED-FF)
LS = labor share index, 2009 base year
NX = net exports
rGDP = real GDP
CPIall = year over year % change of CPI for all items.
ED = policy rate prescribed by Effective Demand Monetary rule
FF = Fed Funds rate
This limit function (L) is then entered in the effective demand equation…
Effective demand limit, EDL = rGDP*a*T/L (1 -(1 – 1/a)*T/L)
T = TFUR, capacity utilization * (1 – unemployment rate)
a = coefficient, set at 3.
How can you do this equation at home? You first set the coefficients for the variables LS, NX/rGDP and CPIall. This gives you a baseline for the limit function. Then you add this baseline into the Effective Demand monetary rule (ED) to evaluate the reaction function of monetary policy (FF) to the baseline economic conditions. Then you feed back (ED-FF) into your limit function and adjust the coefficient on (ED-FF).
So you have to use a monetary rule based on the limit function (L) and then compare it to the actual Fed rate. Since I am the only economist currently with a monetary rule based on a this type of limit function to the utilization of labor and capital (TFUR), an economist would have to use my Effective Demand Monetary rule to crunch the numbers. They would not work in the Taylor rule, unless it was modified.
Some Reflections
It seems the large overshoot of effective demand in 1973 reflects the extent to which the economy was taken by surprise with higher commodity prices, such as fertilizer from Peru and oil from OPEC. Inflation began to rise briskly due to that overshoot. Production was not cut in an attempt to hold down inflation apparently. And even though there was another supply shock later in the 70′s, we do not see an overshoot of effective demand due to a response that raised effective demand by combining a higher labor share with a much more accommodative monetary policy.
At this point, this new equation is saying that there is currently spare capacity still available to the economy and that the unemployment rate would go down to 5.5%. When we eventually see the top of this business cycle, we will have another data point to refine the coefficients in the limit function. Still, the equation is showing a zone where the utilization of labor and capital (TFUR) will hit a limit.
Going forward… the limit function tells us that there are various ways to extend the present business cycle.
- raise labor share
- keep the Fed rate as low as possible, ZLB
- increase net exports as a share of GDP
- keep inflation low
The equation does not tell us about the risks from pushing the business cycle beyond a balanced state. It only tells us how we can extend or cut short the business cycle. Everything is being done at the present moment to extend the business cycle, except for raising labor share. Is this good? Time will tell…
One final note… Look at how production overshot the effective demand limit between 2005 and 2007 in graph #4. It should not be that tight, otherwise a contraction in production would have occurred earlier. The reason is that long term interest rates did not rise at the same pace as the overnight Fed rate. The reason normally given points to the foreign funds flooding back into the US from countries like China. So monetary policy was not as tight as the limit function is assuming. So the variable (ED-FF) will have to incorporate a measure for the differential between short-term and long-term interest rates.
The equation is not perfect yet, but it is getting better.
>> Continue to New Variable For Effective Demand Limit… Part 3
Disclosure: None.